Essential Maths Skills for A-Level Chemistry: What Every Student Needs to Master
If you treat the maths side seriously—just as you would your organic mechanisms or exam timing—you’ll gain an edge over other students.
If you're studying A-Level Chemistry, you're not just learning about atoms, bonding, and organic reactions—you're also entering the world of applied mathematics.
Roughly 20–30% of the marks in A-Level Chemistry papers come from maths-based questions. That’s more than in GCSE and far more than most students expect at first. These marks can make the difference between a B and an A, or an A and an A*—especially in quantitative-heavy topics like thermodynamics, kinetics, and equilibrium.
This guide breaks down the essential maths skills you need, how they apply to each part of the course, and how to practise them to ensure confidence in every calculation.
Why Maths Matters in A-Level Chemistry
You’ll be expected to:
Use and rearrange formulae fluently
Understand and interpret logarithmic and exponential functions
Calculate gradients and rates from graphs
Apply ratios, proportions, and standard form
Handle significant figures, units, and uncertainty correctly
Analyse numerical results in context (not just crunch numbers)
Unlike in GCSE, marks are awarded for your thinking process, not just your final answer. You’ll need to show clear, logical steps.
Let’s explore the key maths skills topic by topic.
1. Rearranging Equations
Whether it's moles = mass ÷ Mr or Kc = [products]/[reactants], you’ll frequently need to rearrange formulae.
Example:
If ΔG = ΔH – TΔS, rearrange to make T the subject:
T = (ΔH – ΔG) ÷ ΔS
Top Tips:
Practise rearranging both linear and more complex formulae
Use bracket logic carefully when substituting
Always write units with your final answer
2. Moles, Mass, and Concentration
At A-Level, moles calculations get more sophisticated:
Solutions: mol = concentration × volume (mol/dm³ × dm³)
Gases: use the ideal gas equation
Stoichiometry: mole ratios become critical in mechanisms and redox titrations
Example:
What volume of 0.2 mol/dm³ NaOH is needed to neutralise 25.0 cm³ of 0.1 mol/dm³ HCl?
moles of HCl = 0.1 × 0.025 = 0.0025 mol
Ratio NaOH:HCl = 1:1
volume NaOH = 0.0025 ÷ 0.2 = 0.0125 dm³ = 12.5 cm³
3. Working with Units and Conversions
You’ll need to be fluent with:
cm³ to dm³ (÷1000)
g to kg, J to kJ, Pa to kPa
dm³ to m³ (for the gas equation)
converting units in enthalpy, equilibrium, and electrochemical problems
Example:
When using PV = nRT, volume must be in m³ and pressure in Pa.
4. Thermodynamics and Enthalpy Calculations
Includes:
Q = mcΔT
ΔH = –Q ÷ n
Hess’s Law cycles
ΔG = ΔH – TΔS
Calculating entropy (J/mol·K) and converting to kJ when necessary
Example:
Q = 100 × 4.18 × 10 = 4180 J
Convert to kJ = 4.18 kJ
🔥 You’ll often need to justify the feasibility of a reaction using ΔG calculations—linking numerical data with theory.
5. Kinetics and Rate Calculations
You’ll need to:
Plot and interpret rate–concentration graphs
Calculate orders of reaction
Determine the rate constant (k) using data and graphs
Use logarithmic methods in the Arrhenius equation
Key Formulae:
rate = k[A]^m[B]^n
k = rate ÷ [A]^m
ln k = –Ea/R × (1/T) + ln A
Skills:
Find gradients
Use ln and e functions on a calculator
Rearranging log equations to find activation energy
6. Equilibrium and Kc/Kp Calculations
You’ll encounter:
Kc = [products]ⁿ / [reactants]ⁿ
Use of ICE (initial-change-equilibrium) tables
Kp = p(products)ⁿ / p(reactants)ⁿ (partial pressures)
Example:
In an equilibrium problem:
calculate moles
divide by volume to find concentrations
apply Kc formula precisely
always include units if required
7. Graph Interpretation and Gradient Calculations
Used in:
Reaction rates
Arrhenius plots
Titration curves
Energetics (enthalpy vs. time)
Key Skills:
Estimate gradients (rise/run)
Interpret trends and curvature
Calculate intercepts for ln k = –Ea/R × 1/T graphs
8. Electrochemical Cells and Ecell Calculations
Key formula:
Ecell = E°(reduction) – E°(oxidation)
Also:
Applying standard electrode potentials
Justifying redox feasibility
Interpreting electrochemical series data
⚡ You’ll often need to analyse whether a reaction is feasible, based on the sign and value of Ecell.
9. Significant Figures and Decimal Places
These appear in:
All numerical answers
Data analysis questions
Practical calculations
Exam Tip:
If data is to 3 sig. figs., your final answer must also be to 3 sig. figs. unless told otherwise.
10. Practical Chemistry and Error Analysis
Includes:
Percentage error
Uncertainty in measurements
Error propagation
Repeatability and reproducibility
Formula:
% error = (uncertainty ÷ reading) × 100
⚠️ You’ll often be asked to explain how to reduce error—e.g., use more sensitive apparatus or increase volume measured.
Study Tips for Mastering A-Level Chemistry Maths
1. Build a Formula Reference Sheet
Keep a single page with all the equations—ideal for quick review before past paper sessions.
2. Practise Topic-by-Topic
Use resources like Physics & Maths Tutor, Save My Exams, and exam board-specific questions to isolate skills.
3. Focus on Mark Scheme Wording
Use the exact scientific phrasing expected, especially in written justifications.
4. Show All Working
Even if your final answer is wrong, clear method steps can earn partial marks.
5. Don’t Panic—Break It Down
Tackle multi-step questions calmly. Draw diagrams, underline given values, and set up the solution logically.
A-Level Chemistry Formula Sheet Summary
A-Level Chemistry Formula Sheet Summary
Final Thoughts: Own the Numbers, Own the Grade
A-Level Chemistry is a subject where understanding and calculation go hand in hand. If you treat the maths side seriously—just as you would your organic mechanisms or exam timing—you’ll gain an edge over other students.
Maths doesn’t have to be a weakness. With the right approach, it becomes your secret weapon.
Need Support With A-Level Chemistry Calculations?
Dr. Marguerite Quinn (PhD Chemistry, MEd) offers specialist online tutoring designed to build confidence and exam success—especially in quantitative topics.
👉 Book a 15 mins consultation to explore how structured 1:1 lessons can help you master every formula, calculation, and graph that comes your way.



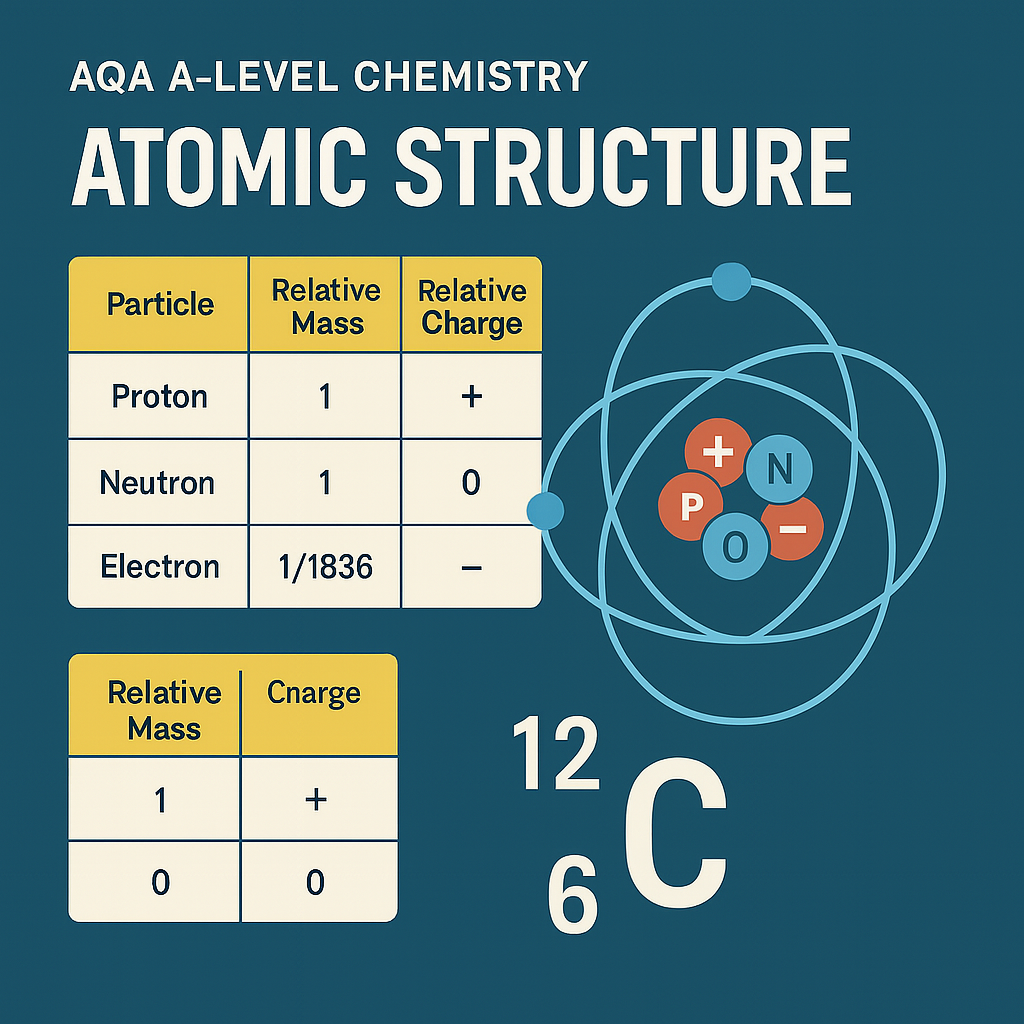


Understand AQA A-Level Chemistry Section 3.1.1.2 on mass number and isotopes. Learn key definitions, isotope notation, calculations, and how this topic builds your scientific and exam skills.